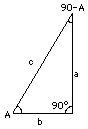
You already know that right angled triangles are basic to trigonometry. Let ABC be such a triangle (drawing) with C = 90° the right angle and (A, B) the sharp ("acute") angles. Also, let a, b and c be the lengths of its three sides--a of the side across from A, b of the one across from B, and c the longest one, across from C. Two useful ratios associated with the angle A ("trigonometric functions of A") are
Both these ratios involve the long side c ("hypotenuse" in mathspeak), and since both a and b must be smaller than that side, these ratios are always numbers less than 1. We now add two more ratios to our collection--the tangent and the cotangent: The tangent of A And the cotangent of A
Multiply top and bottom by c (it is the same as multiplying the fraction by (c/c)=1) and get
The calculators which give sines and cosines, and the books which tabulate their values, are also able to give tangents and cotangents.
A Simple ApplicationAt noon a vertical flagpole of height 50' (50 feet) has a shadow of length 18 feet. What is the angle A of the Sun above the horizon? (As explained in the section "Navigation, " that angle allows one to calculate the latitude at the location.) From the drawing:
If you have a table of tangents, you can now look for the angles whose tangents just above and just below that value, and estimate where between them A should be ("interpolate"). Calculators usually have a button "tan" which, if you punch in the angle, gives you the tangent. But many also have a button "tan -1 " which does the reverse--given the tangent, it brings up the angle. (It may be the same button, deriving tan-1 if first you hit a colored "special mode" button; tan-1 is also called "inverse tangent" or "arc-tangent"). In this example,
P.S.: A tangent to a circle is a line grazing it at just one point. If you ever wondered how the name "tangent" entered trigonometry, click here. |
 A simple relation exists between this pair and the earlier ones. We have
A simple relation exists between this pair and the earlier ones. We have