|
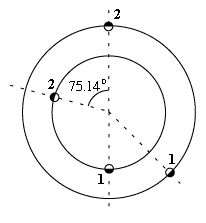
(0.70873) (360) = 255.140
It will therefore reach position "2" on the inner circle, 75.140 past the position of Mars. Notice how Earth has overtaken Mars--at launch (position "1") it lagged behind, but now it is ahead. As Kepler's 3rd law shows, the closer a planet is to the Sun, the faster it completes its orbit, and Earth is closer than Mars.
Suppose the spacecraft which has landed on Mars is a robot , which collects a sample and immediately takes off again for its return trip. Launching from point (2), after breaking free from the planet's gravity, it can again follow the Hohmann transfer ellipse, in a mirror image of the flight to Mars (drawing below).
Its trip starts with a reverse thrust of 2.545 km/s, reducing its orbital velocity from V3 to V2. Then, after 0.70873 years, it arrives again at the point marked (1) with velocity V1, which needs to be reduced to the Earth's orbital velocity V0 by a reverse thrust of 2.966 km/s. Unfortunately ... Earth will not be waiting there!
The Synodic Period
|
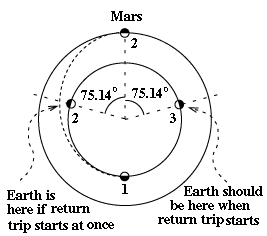
| Mars and Earth at the start of the return trip
|
Let's see where Earth should be at the time of launch from Mars, in order for the return flight to meet it at the proper time.
The return trip, being half the Hohmann ellipse, takes 0.70873 years, during which (as calculated above) Earth covers an arc of 255.140 in its orbit. To meet the return rocket when it reaches Earth's orbit at point (1), Earth must be--at the start of the return flight from Mars --255.140 behind point "1" in its orbit. That puts it at position (3), 75.140 behind the position of Mars, not at position (2) where Earth is 75.140 ahead of Mars.
Since Earth and Mars constantly change their relative position, it stands to reason that if we sufficiently delay the return trip, Earth will move from position (2) relative to Mars to position (3), at which time the return trip can begin.
Let us calculate that delay. To simplify the calculation of the delay, we calculate the relative rotation velocity between Earth and Mars around the Sun.
- The rotation velocity of Earth is 1 orbit per year.
- The rotation velocity of Mars is 1 orbit per 1.8822 years, i.e.
1/1.8822 = 0.531293 orbits per year.
Each year, Earth increases its lead over Mars by (1 - 0.531293) = 0.468707 orbits
In 2 years, the lead is (2)( 0.468707) = 0.937414 orbits
and Earth will be one full orbit ahead after
1 / 0.468707 = 2.13353 years
If Mars and Earth start out on the circle abreast of each other, after 2.13353 they will be again abreast. From the point of view of an observer on Earth, that is the time required by Mars for one full circle around the sky. It is known as the synodic period of Mars, and is about 25.6 months.
The rest is easy. For the Earth to change its position relative to Mars from (2) to (3), Earth must advance (relative to Mars) by
360 - (2)(75.14) = 209.720
To advance by 3600 takes 2.13353 years, so to advance by the above angle takes
(209.72 / 360)( 2.13353) = 1.2429 years = 454 days
A more accurate calculation gives 459 days (ours contains approximations). When the return rocket arrives at Earth, it will be overtaking it, since its velocity V1 is exceeds the orbital velocity V0 of Earth by about 3 km/s. Before safely descending to the ground, the spacecraft also must get rid of the velocity v0 given to it by the pull of the Earth, about 11.3 km/s. However, if it reenters by skimming the atmosphere "just right," its extra kinetic energy will safely dissipate as heat, with no need for more rocket firings.
Afterthoughts
Our diagrams only mark progress in circular orbits around the Sun, which keep approximately the same speed. The relative motion of Earth and Mars in the sky is much more variable, because the Earth-Mars distance changes all the time. Indeed, when Earth is closest to Mars and overtaking it, Mars will seem (for a while) to be moving backwards among the stars. The total period however remains 25.6 months, quite different from the actual orbital period of Mars which is 1.8822 years = 22.6 months.
Considering all those complications, one can appreciate the subtlety of the work of Copernicus and Kepler, who extracted clean regular patterns of motion, from the much less regular ones of planets across the heavens.
Questions from Users:
Overtaking planets
***
A "short stay on Mars"
|