In contrast, Newton's 3rd law always involves more than one object.
- When a gun is fired, the force of the gas produced by burning gunpowder hurls out the bullet. By Newton's law, the gun itself recoils backwards.
- The nozzle of a big firehose has handles which firefighters must grasp firmly, because as the jet of water shoots out of it, the hose itself is forcibly pushed back.
- Rotating garden sprinklers work by the same principle. In a similar way, the forward motion of a rocket comes from the reaction of the fast jet of hot gas shooting out from its rear.
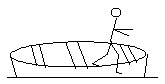
Those familiar with small boats know that before jumping from a boat to the dock, it is wise to tie the boat to the dock first, and to grab a handhold on the dock before jumping. Otherwise, even as you jump, the boat "magically" moves away from the dock, possibly making you miss your leap or pushing the boat out of reach. It is all in Newton's third law: as your legs propel your body towards the dock, they also apply to the boat an equal force in the opposite direction, which pushes it away from the dock.
The Bicycle
 A more subtle example is afforded by the bicycle. It is well known that balancing a bicycle standing still is almost impossible, while on a rolling bike it is quite easy. Why?
A more subtle example is afforded by the bicycle. It is well known that balancing a bicycle standing still is almost impossible, while on a rolling bike it is quite easy. Why?
Different principles are at work in each case. Suppose you sit on a bike that stands still, and find it is leaning to the left. What do you do? The natural tendency is to lean to the right, to counterbalance the lean with your weight. But in moving the top of your body to the right, by Newton's 3rd law you are actually pushing the bike to lean more to the left. Maybe you should lean to the left and push the bike back? It might work for a fraction of a second, but now you are really out of balance. No way!
On a rolling bike, balance is kept by a completely different mechanism. By slightly turning the handlebars right or left, you impart some of the rotation of the front wheel ("angular momentum") to rotate the bike around its long axis, the direction in which it rolls. That way the rider can counteract any tendency of the bike to topple to one side or the other, without getting into the vicious circle of action and reaction.
To discourage thieves, some bikes contain a lock which clamps the handlebars in a fixed position. When such a bike is locked in the forward-facing direction, it can be rolled by a walking person, but it cannot be ridden because it cannot be balanced.
(Optional Addition)
Mach's formulation of Newton's laws
 |
| Ernst Mach |
Newton's laws were introduced here in the traditional way--through the concepts of mass and force (Newton actually formulated the second law in terms of momentum, not acceleration). Ernst Mach, who lived in Germany two centuries after Newton, tried to avoid new concept and formulate physics only in terms of what can be observed and measured. He argued that Newton's laws boil down to one law:
"When two compact objects ("point masses" in phystalk) act on each other, they accelerate in opposite directions, and the ratio of their accelerations is always the same. "
Read it again, if you will: no mention of force or mass, only of acceleration, which can be measured. When a gun acts on a bullet, a rocket on its exhaust jet, the Sun on Earth (and on the scale of the distance separating the two, Sun and Earth can be viewed as compact objects), the accelerations are always oppositely directed.
Mass and force are now readily derived. If one of the objects is a liter of water, its mass is defined as one kilogram. If it then acts on another object (perhaps with the water frozen into ice, for the purpose of the experiment), then the ratio of its acceleration aw to the acceleration a of the other object gives the object's mass m. And a force of 1 newton is defined as the one that causes 1 kg an acceleration of 1 m/sec.
Questions from Users:
Question about balancing a bicycle
|

 A more subtle example is afforded by the bicycle. It is well known that balancing a bicycle standing still is almost impossible, while on a rolling bike it is quite easy. Why?
A more subtle example is afforded by the bicycle. It is well known that balancing a bicycle standing still is almost impossible, while on a rolling bike it is quite easy. Why?